`\color{green} ✍️ ` The plane having a complex number assigned to each of its point is called the `color(blue)"complex plane or the Argand plane."`

`\color{green} ✍️ ` We already know that corresponding to each ordered pair of real numbers `(x, y),` we get a unique point in the `XY` plane and vice-versa with reference to a set of mutually perpendicular lines known as the x-axis and the y-axis.

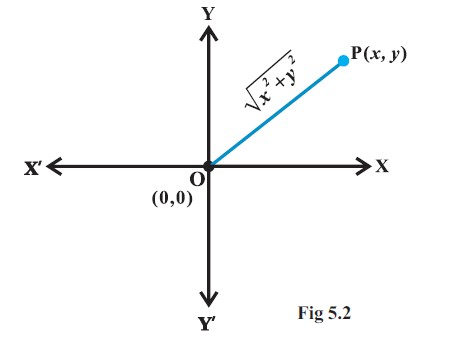
`\color{green} ✍️ ` The complex number `x + iy` which corresponds to the ordered pair `(x, y)` can be represented geometrically as the unique point `P(x, y)` in the `XY`-plane and vice-versa.

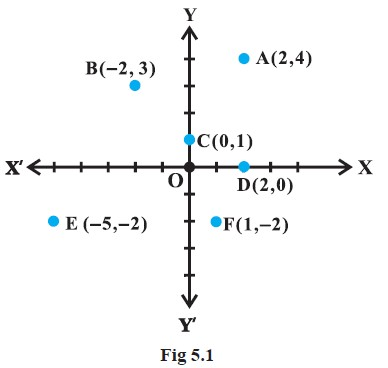
`\color{green} ✍️ ` Some complex numbers such as `2 + 4i, – 2 + 3i, 0 + 1i, 2 + 0i, – 5 –2i` and `1 – 2i` which correspond to the ordered pairs `(2, 4), ( – 2, 3), (0, 1), (2, 0), ( –5, –2),` and `(1, – 2),` respectively, have been represented geometrically by the points `A, B, C, D, E,` and `F,` respectively in the Fig 5.1.

`\color{green} ✍️ ` The points on the `x`-axis corresponds to the complex numbers of the form `a + i 0`
and the points on the `y`-axis corresponds to the complex numbers of the form `0 + i b.`

`\color{green} ✍️ ` The `x`-axis and `y`-axis in the Argand plane are called, respectively, the `color(blue)"real axis"` and the `color(blue)"imaginary axis."`

`\color{green} ✍️ ` `color(blue)(|x + iy| = sqrt(x^2 + y^2))` is the distance between the point `P(x, y)` to the origin `O (0, 0)` (Fig 5.2).

`\color{green} ✍️ ` The representation of a complex number `color(blue)(z = x + iy)` and

its conjugate `color(blue)(z = x – iy)` in the Argand plane are, respectively, the points `color(blue)(P (x, y))` and `color(blue)(Q (x, – y)).`

`\color{green} ✍️` Geometrically,
`color(blue)("the point (x, – y) is the mirror image of the point (x, y) on the real axis")` (Fig 5.3).

`\color{green} ✍️` Let the point `P` represent the nonzero complex number `color(blue)(z = x + iy.)`

`\color{green} ✍️` Let the directed line segment `OP` be of length `r` and `θ` be the angle which `OP` makes with the positive direction of `x`-axis (Fig 5.4).

`\color{green} ✍️` We may note that the point `P` is uniquely determined by the ordered pair of real numbers `color(blue)((r, θ)),` `color(red)("called the polar coordinates of the point P.")` We consider the origin as the pole and the positive direction of the `x` axis as the initial line.

`\color{green} ✍️` We have, `color(blue)(x = r cos θ, y = r sin θ)` and therefore, `color(blue)(z = r (cos θ + i sin θ).)` The latter is `color(red)("said to be the polar form of the complex number. ")`

`\color{green} ✍️` Here `color(blue)(r = sqrt(x^2 + y^2) = |z|)` is the modulus of `z` and `θ` is called the argument (or amplitude) of `z` which is denoted by `arg z.`

`\color{green} ✍️` For any complex number `z ≠ 0,` there corresponds only one value of `θ` in `0 ≤ θ < 2π.` However, any other interval of length `2π,` for example `– π < θ ≤ π,` can be such an interval.

`\color{green} ✍️` We shall take the value of `θ` such that `color(red)(– π < θ ≤ π),` called `color(red)("principal argument of z")` and is denoted by `arg z .` (Figs. 5.5 and 5.6)